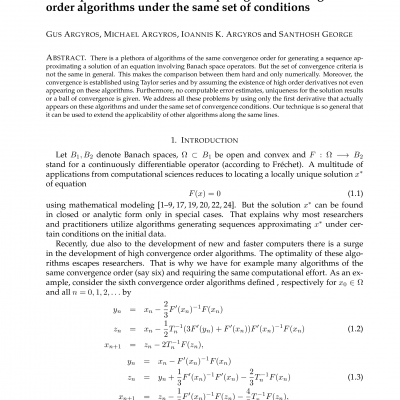
The relation between integer powers of the generalized Fibonacci matrix
and generalized Fibonacci numbers is well known, where 
and 
are nonzero real numbers. Inspired by this relation, a procedure is presented to find some 
dimensional nonsingular matrices whose powers are related to generalized Fibonacci numbers.
Some 3×3 dimensional nonsingular matrices related to generalized Fibonacci numbers
Petik, Tuğba, Akbulut, Hilal and Özdemir, Halim
Full PDF
creative_2021_30_1_81_88Additional Information
| Author(s) | Petik, Tuğba, Akbulut, Hilal, Özdemir, Halim |
|---|
Recently posted
-
Uniqueness of Shift Polynomials and Derivative Sharing Polynomial
-
Diffeomorphic embedding of Higher-dimensional Hilbert Manifolds into Hilbert spaces
-
Approximating fixed points of the SP*-iteration for generalized nonexpansive mappings in CAT(0) spaces
-
Leverage Centrality in Corona Product of Cycle with some Graphs
-
Some new results on WS-algebras
-
On Duo Γ-semihyperrings
-
Comparative Study of Different Vacation Schemes in an Inventory System with Production and Multiple Servers
-
Isomorphism of Line Graph and Common Neighbourhood Graph
-
Timelike Bonnet surfaces on Lorentzian three-manifolds
-
Nonassociative van der Waerden rings